A sensitive, frequency-modulated dip meter capable of measuring resonator Q

Abstract

A highly-sensitive, narrow-band frequency-modulated dip meter offers a simple way to measure resonator Q.

Background

I have been working with an unusual dip meter circuit recently that has some interesting properties.

The dip meter, also called a grid dip meter, is a simple homebrew instrument that offers a wide range of measurement possibilities. For an introduction to dip meters, please see https://hackaday.com/2015/11/30/the-grid-dip-meter-forgotten-instrument/ and https://www.robkalmeijer.nl/techniek/electronica/radiotechniek/hambladen/qst/2002/05/page65/index.html .

Problem with existing dip meters: low sensitivity

The problem with most dip meters is low sensitivity. A strong magnetic coupling to the device under test is required to obtain a sufficiently-large dip to be noticed. On the other hand, strong coupling has the disadvantage that it detunes the oscillator and also loads the device under test, reducing its Q. 

Ideally, the coupling should be as weak as possible to obtain the most accurate result. But with weak coupling, the meter deflection is very small and very difficult to discern.

As an example of low dip meter sensitivity, see the following video. This is a simple and traditional dip meter (GDO), dipping a ferrite rod antenna. A weak dip of a few microamps can be detected. The circuitry surrounding the ferrite rod forms a superheterodyne receiver, which is turned off and is irrelevant for this test. At the end of the video, after the dip is detected, I move the dip meter physically farther from and closer to the coil, to indicate how the dip meter output changes with distance to the coil being dipped. 

https://www.youtube.com/watch?v=iK8aZhySox8

The dip is very small, and the movement of the needle is very difficult to discern on the meter.

The circuit for this dip meter is from the webpage of Mr. B. Kainka at http://www.b-kainka.de/bastel53.htm. The signal that moves the meter is a DC signal derived from the RF oscillator's voltage. Because this is a DC signal, it is more complicated to amplify than an AC signal.

Improved dip meter sensitivity: Narrow-band frequency modulation generates easy-to-amplify AC signal

One good solution to improving dip meter sensitivity is to introduce a small frequency modulation onto the dip meter's RF oscillator. The dip meter frequency repeatedly is swept up and down by several kHz around the currently-tuned frequency of the dip meter. The sweep frequency is at an audio frequency such as 500 Hz.

This repeated, narrow-band frequency modulation then generates a small 500 Hz AC signal that corresponds to the variation in oscillator amplitude inside of the narrow-band sweep. If the oscillator amplitude does not vary, or varies only slightly, inside of this few-kHz sweep width, then no signal or only a very small AC signal is generated. On the other hand, if the oscillator amplitude starts to change rapidly inside of the few-kHz sweep width -- as will be the case when the dip meter is tuned to a frequency where a dip is present -- then a large 500 Hz AC signal is generated. What is actually happening is that we are measuring the rate of change of the resonance curve of the device under test. The greater the rate of change, the greater the oscillator amplitude variation inside of the repeating narrow-band frequency sweep, and the greater the amplitude of the generated 500 Hz AC signal.

The generated 500 Hz AC signal -- since it is AC and not DC -- can then easily be amplified by a normal AF amplifier such as an LM386. The amplified audio signal can then be monitored directly via headphones, and can also be used to drive a meter or an LED for visual display.

To my knowledge, the first and only commercial dip meter to use this concept is the DipIt meter, described here: https://www.qrpproject.de/UK/DipItUk.html. (Edited 2024/07 -- link seems dead; here is an alternative link: https://www.qsl.net/sp5btb/DipIt.pdf .)

However, the concept of frequency-modulating the dip meter is not new. As far back as 1972, the technique was already fully described within the context of nuclear quadropole resonance experiments by M. Suhara at http://scirep.w3.kanazawa-u.ac.jp/articles/17-01-002.pdf. 

Quote (emphasis added):

In  magnetic resonance experiment，the nuclear signal can be detected through modulations; one is frequency modulation and other magnetic field modulation (or Zeeman modulation). In principle, the use of frequency modulation of the source oscillator of a radio-frequency spectrometer offers an attractive mode of operation. As the frequency of the spectrometer oscillator moves back and forth in the region of an absorption line of the sample, the latter produces amplitude modulation, which can be observed by means of an amplitude detector, followed by narrow-band amplification and phase-sensitive detection at the modulation frequency.

Because the frequency-modulation technique was already fully-described in 1972, it is likely that the technique was already known several years earlier.

My frequency-modulated dip meter circuit

My dip meter circuit is as follows.

The principle of operation is described on the schematic image and requires no further explanation here.

Here is a video of my frequency-modulated dip meter in use.

https://www.youtube.com/watch?v=nVi3L0BwaXg

In the above video, the new, frequency-modulated dip meter is used to detect a dip in the same configuration as the previous video with the traditional (non-frequency-modulated) dip meter. The new circuit clearly shows much better sensitivity. A strong dip is indicated by a loud audio tone and bright LED illumination. At the end of the video, after the dip is detected, I move the dip meter physically farther from and closer to the coil, to indicate how the dip meter outputs (audio volume and LED brightness) change with distance to the coil being dipped.

Measuring Q with a traditional (non-frequency-modulated) dip meter

It is probably well-known that a dip meter can be used to measure Q of an inductor or resonant tank. See for instance https://www.robkalmeijer.nl/techniek/electronica/radiotechniek/hambladen/qst/2002/05/page65/index.html. You essentially tune the dip meter for a deep dip, then slowly tune the meter to either side of the dip until the dip depth is reduced by 30%, that is, such that the dip is only 70% of its original value. Then, the frequency difference between the two 70%-dip frequencies is the -3 dB bandwidth. In other words, as the oscillator is tuned across the dip, the dip in oscillator amplitude basically follows the shape of the resonance curve of the device under test.

This method is already attractive because it requires no direct connection to the device under test. But the problem with this approach is that it requires a rather high level of inductive coupling to the resonator to get a deep dip. Such high coupling can cause unwanted loading of the device under test. Also, it is difficult and tedious to identify, from meter readings, when the meter value corresponds to 70% of its maximum deflection from the baseline value. It is even more difficult when the coupling is reduced to a minimum, which also then reduces the meter deflection to a minimum.

Measuring Q with a narrow-band frequency-modulated dip meter

It was mentioned above that with a narrow-band frequency-modulated dip meter, we are not measuring the resonator response of the device under test, but instead we are measuring the rate of change -- the first derivative -- of the resonator response.

Therefore, when manually tuning a narrow-band frequency-modulated dip meter across the resonance frequency of a resonator being tested, you get a null (a zero rate of change) at the exact center resonance frequency. You also get two peaks, one on each side of the resonance frequency -- these peaks correspond to the frequencies where the slope (the rate of change of the resonance curve) is maximum.

It turns out that at the frequencies where these two peaks occur -- at the frequencies where the slope of the resonance curve is maximum -- the amplitude of the resonance curve has dropped to 75%. This is proved by finding the zero-crossing points of the second derivative of a Cauchy-Lorentz function that mathematically models the resonance response (see https://en.wikipedia.org/wiki/Cauchy_distribution). I plan to write a summary of the derivation and post it here on my blog in the future, but the basic idea is that the zero-crossings of the second derivative show the location of the maximum/minimum points of the first derivative (and the first derivative is what we measure with the frequency-modulated dip meter). If we take the algebraic expressions for frequencies where these second-derivative zero-crossings occur, and use those frequencies to evaluate the value of the original Cauchy-Lorentz function (the resonance response function) in relation to the maximum value of the Cauchy-Lorentz function, it turns out that the relationship is that the value is always 75% of the maximum value.

In other words, the frequency difference between the dual peaks that are measured on a narrow-band frequency-modulated dip meter corresponds to a bandwidth where the resonator response has reduced to 75% of its maximum. This corresponds to a -2.498 dB bandwidth, which can (with some more pages of mathematical derivation) be converted to a -3 dB bandwidth by multiplying by a scaling factor of 1.1147.

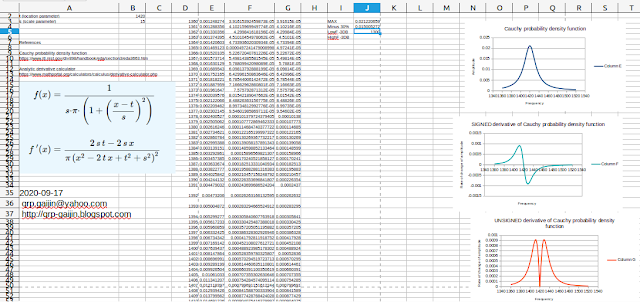
Below is an image showing a resonance curve, its first derivative, and the absolute value of its first derivative (lower right). The lower-right image corresponds to the output data that would be obtained from a narrow-band frequency-modulated dip meter that is tuned across the resonance peak of a device under test.

 

Conclusion

This new kind of dip meter -- a narrow-band frequency-modulated dip meter that measures the first derivative of the resonance curve, and that generates an AC output signal that can be easily amplified and thus leads to good sensitivity -- potentially offers an easy way to measure resonator bandwidth (and hence Q) with minimal loading of the device under test. All that is needed is some quite light magnetic coupling to the inductor under test.

The special thing about the new kind of narrow-band frequency-modulated dip meter is that its sensitivity is increased, and it offers two unambiguous peaks that serve as "markers" of where the resonator response has dropped to 75% of its maximum. This should make bandwidth measurement easier as compared with traditional (non-frequency-modulated) dip meters.

I should add that I am not an expert on Q measurements, but I am very intrigued by the ability of this new dip meter design to fairly easily measure inductor Q. I do not have other test equipment capable of measuring resonator Q, but I hope that some other experimenters with better test equipment might be encouraged to build this kind of a new dip meter and to compare the bandwidth and Q measurements from the dip meter (bandwidth = 1.1147 * frequency difference between the two peaks; then Q=frequency/bandwidth) with the Q measurements obtained by other means.

Of course, the whole idea of using a dip meter to measure resonator bandwidth is quaint and outdated in this day and age of $50 vector network analysers. But it's much more fun and educational (for me anyway) to use traditional and hands-on methods, instead of punching some buttons and reading the result from a computer screen.